Validation Case: Thick Plate Under Pressure

This validation case belongs to pressure load in solid mechanics. The aim of this test case is to validate the following parameters:

• Distributed pressure
• Nodal stress

The simulation results of SimScale were compared to the numerical results presented in [NAFEMS]\(^1\).

Geometry

The geometry used for the case is as follows:

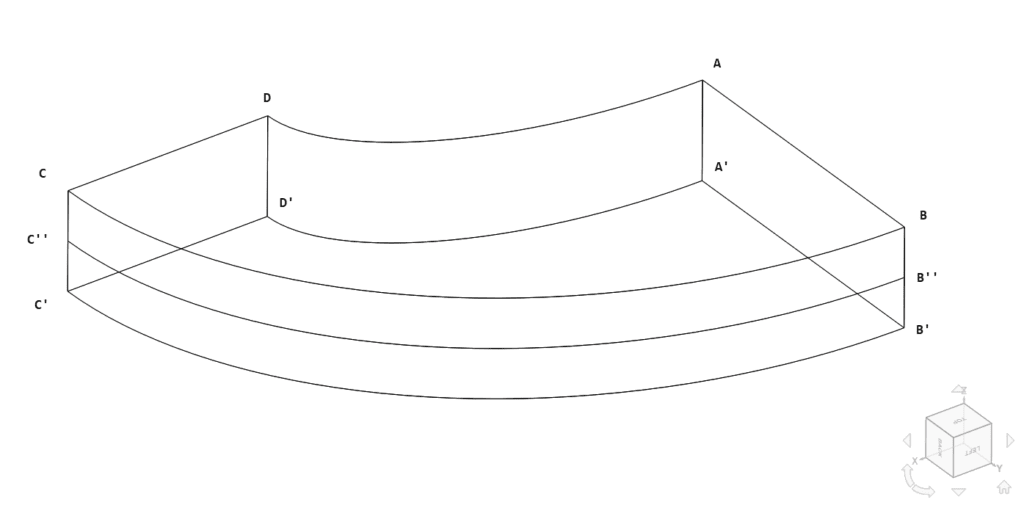
The plate has a thickness of 0.6 \(m\). Table 1 presents the coordinates for each point:

Point	X [\(m\)]	Y [\(m\)]	Z [\(m\)]
A	0	1	0.6
B	0	2.75	0.6
C	3.25	0	0.6
D	2	0	0.6
A’	0	1	0
B’	0	2.75	0
C’	3.25	0	0
D’	2	0	0

The point B” is located at the middle of edge BB’ while point C” is located at the middle of edge CC’. The curves of edges BC and AD are defined by equations 1 and 2 respectively:

$$ ( \frac{x}{3.25} )^2 + ( \frac{y}{2.75} )^2 = 1 \tag{1} $$

$$ ( \frac{x}{2} )^2 + y^2 = 1 \tag{2} $$

Analysis Type and Mesh

Tool Type: Code_Aster

Analysis Type: Static Linear

Mesh and Element Types:

All meshes were computed locally and uploaded to the platform. Table 2 presents the details of the meshes used for each case:

Case	Mesh Type	Number of Nodes	Element Type
A	1st Order Tetrahedral	26639	Standard
B	2nd Order Tetrahedral	194237	Standard
C	1st Order Hexahedral	36	Standard
D	2nd Order Hexahedral	111	Standard
E	1st Order Hexahedral	105	Standard
F	2nd Order Hexahedral	349	Standard
G	2nd Order Hexahedral	52941	Standard

Simulation Setup

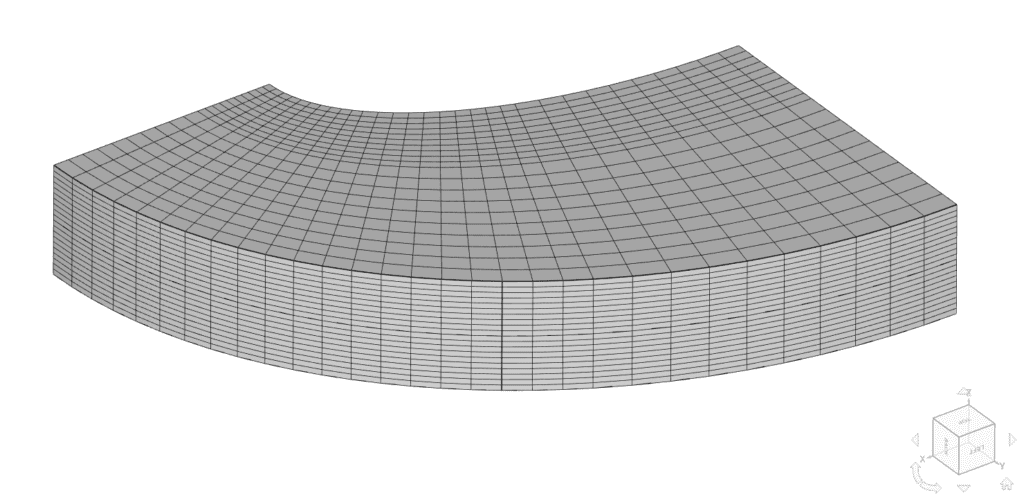
Material:

• Linear Elastic Isotropic:
  • \( E = \) 210 \( GPa \)
  • \( \nu = \) 0.3

Boundary Conditions:

• Constraints:
  • Face DCD’C’ with zero y-displacement
  • Face ABA’B’ with zero x-displacement
  • Face BCB’C’ with zero y- and x-displacements
  • Edge B”C” with zero z-displacement
• Loads:
  • Pressure of 1 \(MPa\) applied on face ABCD

Reference Solution

The reference solution as presented in [NAFEMS]\(^1\) is of the numerical type. For the comparison purposes, the normal stress at point D in the direction of the Y axis is taken:

\( \sigma_{ref} = 5.38\ MPa \)

Result Comparison

Comparison of stress \(\sigma_{yy}\) magnitude computed at the location of point D (variable SIYY) from the simulation results is presented with respect to the reference solution \(\sigma_{ref}\):

CASE	\( \sigma_{ref} \) [MPa]	\( \sigma_{YY} \) [MPa]	Error
A	5.38	5.16166	-4.06 %
B	5.38	5.40075	0.39 %
C	5.38	3.98345	-25.96 %
D	5.38	5.47091	1.69 %
E	5.38	5.10811	-5.05 %
F	5.38	5.71058	6.14 %
G	5.38	5.38599	0.11 %

The von Mises stress color plot is presented for the results of case G:

Related Tutorials

• Linear Static Analysis of a Crane

Last updated: September 4th, 2023