Aerodynamics: Flow around the Ahmed Body

This validation case belongs to fluid mechanics, representing the aerodynamics of the Ahmed body study. The aim of this test case is to validate the following parameters:

• Drag coefficient computation
• Velocity profiles

The simulation results of SimScale were compared to the experimental data presented in [Ahmed]\(^1\).

Geometry

The geometry is created based on the simplified aerodynamic body used by Ahmed et al\(^1\). See Figure 1 for dimensions and Figure 2 for the geometry. The slant angle (\(\phi\)) is set to 25°. The body is placed in a wind tunnel (\(6 m \times 5 m \times 13.5 m\)) in order to limit the aerodynamic blockage effect.

Analysis Type and Mesh

Tool Type: OpenFOAM®

Analysis Type: Turbulent Incompressible fluid flow

Mesh and Element Types:

Mesh #	Mesh Type	Number of Cells	Comments
1	Standard	3,826,602	Far field mesh cell size 0.4 \(m\)
2	Standard	4,673,973	Far field mesh cell size 0.2 \(m\)
3	Standard	8,594,246	Far field mesh cell size 0.1 \(m\)
4	Standard	10,351,196	Body mesh cell size 3 \(mm\)
5	Standard	24,257,180	Body mesh cell size 2 \(mm\)

The Standard Mesher algorithm with tetrahedral and hexahedral cells was used to generate the mesh, with refinements near the walls and in the wake region (see Figure 3).

A typical property of the generated mesh is the \(y^+\) (“y-plus“) value, which is defined as the non-dimensionalized distance to the wall, learn more. A \(y^+\) value of 1 would correspond to the upper limit of the laminar sub-layer.

An average \(y^+\) value of 1 was used for the inflation layer around the body, and 150 for the floor. The \(k-\omega\) SST turbulence model was chosen, with full resolution for near-wall treatment of the flow around the body and with wall function for the floor.

Simulation Setup

Fluid

Air with a kinematic viscosity of \(1.5 \times 10^{-5}\ kg/ms\) is assigned as the domain fluid. The boundary conditions for the simulation are shown in Table 2.

Boundary Conditions

Parameter	Inlet	Top Face	Bottom Face	Lateral Faces	Outlet	Body
Velocity \([m/s]\)	60	Symmetry	Wall Function	Symmetry	Zero Gradient	Full Resolution
k \([m^2/s^2]\)	0.135	Symmetry	Wall Function	Symmetry	Zero Gradient	Full Resolution
\(\omega\) \([1/s]\)	180.1	Symmetry	Wall Function	Symmetry	Zero Gradient	Full Resolution
Pressure \([Pa]\)	Zero Gradient	Symmetry	Wall Function	Symmetry	0	Full Resolution

The free stream velocity of the simulation is \(60\ m/s\), so that the Reynolds number based on the length of the body \(L\) is \(4.29 \times 10^{6} \). Those are the same values presented in the original experiment of Ahmed and Ramm\(^1\).

Reference Solution

The reference solution is of the experimental type, as presented in [Ahmed]\(^1\). It is given in terms of the drag force coefficient:

$$ C_{d} = 0.298 $$

Result Comparison

Drag Coefficient

The drag coefficient is defined as

$$ F_{d}={\frac {1}{2}}\rho \,U^{2}\,C_{d}\,A_x $$

where \(A_x\) (0.115 \(m^2\)) is the projected area of the Ahmed body in streamwise direction and \(F_{d}\) the drag force. The time-averaged drag force was determined by integration of surface pressure and shear stress over the entire Ahmed body. The resulting drag coefficient of the Ahmed body, closest to the reference solution as yielded by the finer mesh (case number 5), was computed to be \(0.304\) which is within a \(1.94%\) error margin of the measured value.

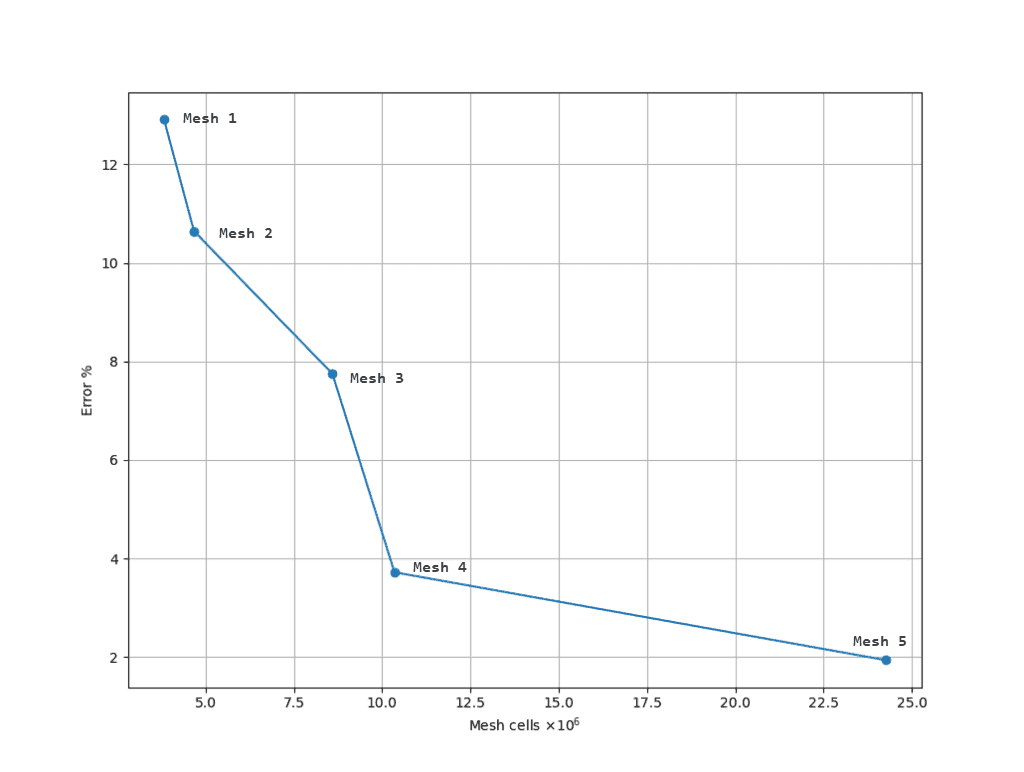
Table 3 shows the result of the mesh independence study:

Mesh #	PRESSURE FORCE \([N]\)	VISCOUS FORCE \([N]\)	TOTAL DRAG \([N]\)	DRAG COEFFICIENT	REFERENCE	ERROR [%]
1	76.758	6.540	83.298	0.336	0.298	12.91
2	74.992	6.637	81.629	0.330	0.298	10.64
3	72.969	6.524	79.493	0.321	0.298	7.75
4	67.671	8.850	76.521	0.309	0.298	3.72
5	64.819	10.387	75.206	0.304	0.298	1.94

Figure 4 shows the mesh convergence plot:

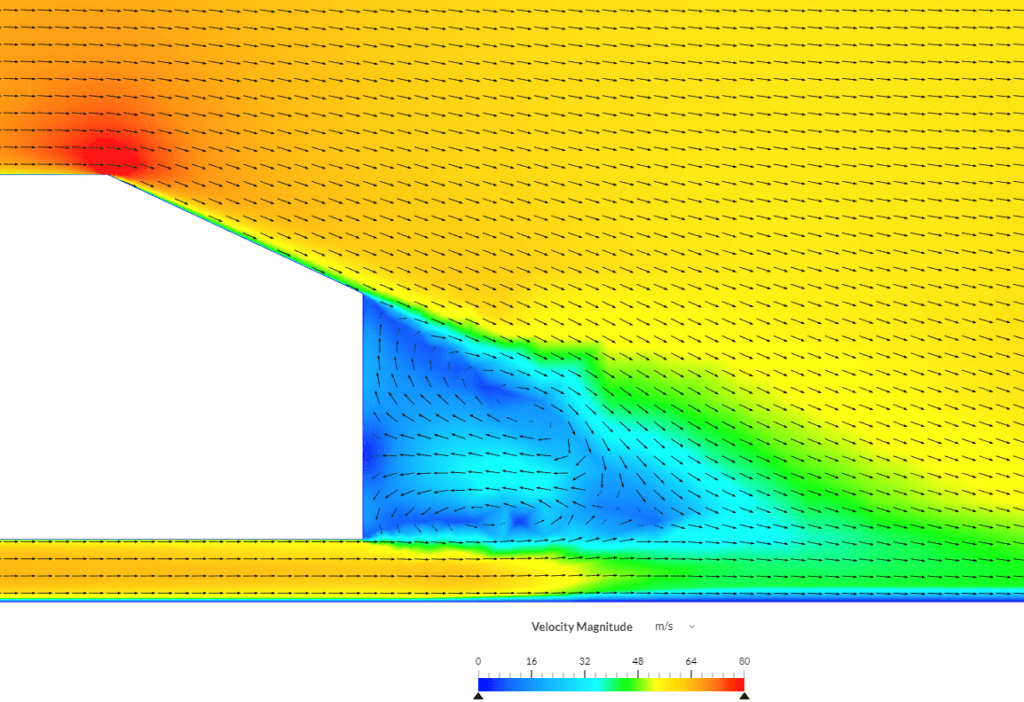
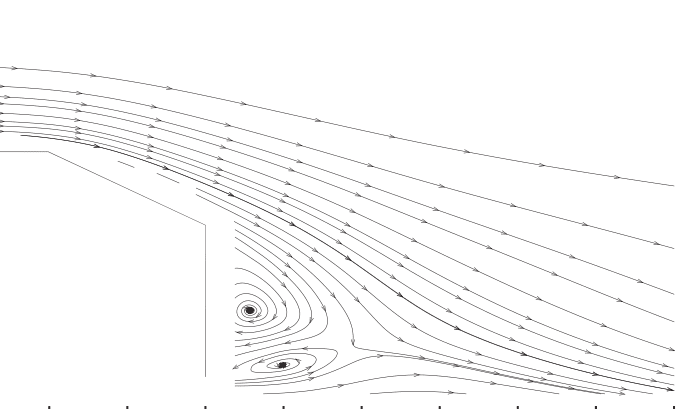
Wake Flow Patterns

The velocity streamline contour of mean flow obtained with the simulation is reported in Figure 5 together with experimental results of reference.

Last updated: December 15th, 2023