Documentation
Radiation is defined as the transfer of energy using electromagnetic waves. When these waves interact with any type of matter, they become heat. All bodies with a temperature greater than absolute zero emit radiation, and in contrast to conduction or convection, this radiation behavior phenomenon requires no medium.
In SimScale, radiation is modeled using a Diffuse View Factors Model. This approximation implies several assumptions that can be applied to most of the engineering problems:
The different surfaces of the model will exchange heat between them, and they will also provide or subtract heat from the adjacent fluid. Coupling of both convection and radiation will be achieved once the simulation converges.
In SimScale, radiation is available for Convective Heat Transfer and Conjugate Heat Transfer v2.0 analysis types and can be activated by toggling on Radiation in the global settings. As a result, you should be able to set radiative behavior, emissivity, additional radiative source, and the far-field temperature while assigning the boundary conditions.
Radiative behaviour specifies the relationship between the net radiative heat per unit surface area, \(Q_r\ [W/m^2]\) and the temperature of every surface, \(T_s\ [K]\). In SimScale, you can set three radiative surface behaviors, opaque, transparent and semi-transparent:
A Transparent surface establishes no relation between \(Q_r\) and \(T_s\). This means that the surface temperature remains unaffected by the net radiative heat that the surface emits or receives. This implies that \(T_s\) is determined by other heat transport means (conduction or convection) or by a boundary condition. This option is mainly applied to surfaces that are not solid, like inlets or outlets (for example, an open window).
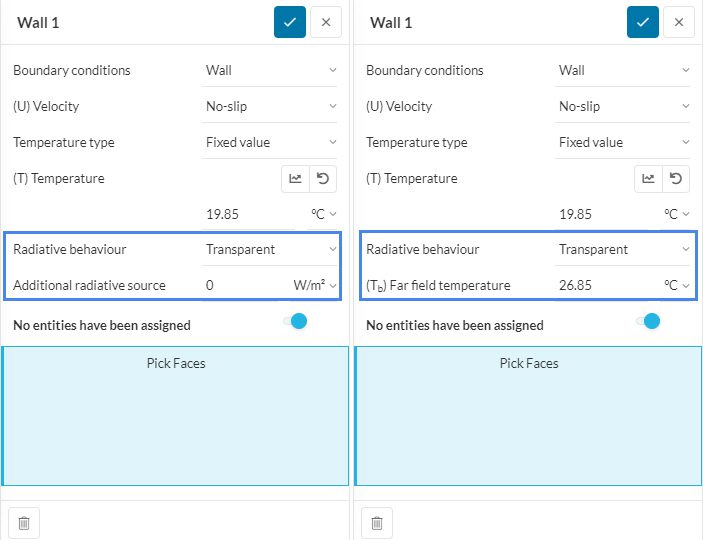
Additional Radiative Source
For convective heat transfer analysis, apart from the radiative heat interchange that the surfaces will perform, we can set an additional radiative source. This represents any additional (mainly external) source of radiation that goes through the surface and it will not heat it up. The best example is solar radiation getting into the domain through an open window.
Far Field Temperature
Available to only conjugate heat transfer v2.0 analysis, far-field temperature represents the temperature of the black body in the far field needed for inclusion of radiation effects at the open boundaries. Hence, instead of mentioning the power of the radiative source you can just mention its temperature.
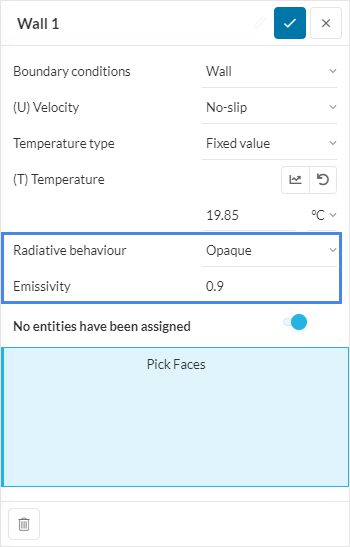
An Opaque behavior couples \(Q_r\) and \(T_s\) using the Stefan-Boltzmann law for diffuse and hemispheric radiation. Supposing two different surfaces \(S\) and \(S’\), the \(Q_r\) that \(S\) provides to \(S’\) is:
$$ Q_r(S→S’) = F. \epsilon . \sigma .(T_S – T_{S’})^4$$
Where \(F\) is the view factor between \(S\) and \(S’\), \(\sigma\) is the Stefan-Boltzmann constant (5.6696e-8 \(W /m²K\)), and \(\epsilon\) is the emissivity of the surface \(S\).
A surface that is not fully transparent is considered a semi-transparent surface. Such a surface partially absorbs, transmits, and reflects the radiation falling on it. The user needs to provide the values for the emissivity as well as transmissivity. This is only available for CHT v2.0 analysis type.
Emissivity
The emissivity depends on the material of the surface, and it measures its capability to emit radiation, otherwise known as its radiation behavior. For opaque surfaces, the default value in the Workbench is 0.9, which is a good approximation for walls made of brick or concrete.
Transmissivity
Transmissivity also depends on the material of the surface, and it measures its capability to transmit radiation. For semi-transparent surfaces, the default value in the Workbench is 0.7, which is a good approximation for stained glass windows.
For radiative heat transfer problems, further settings can be changed within the numerics, as shown in the picture below. Most importantly, the radiation resolution can be changed. This affects the discretization of the directions for which the radiative problem is solved. The settings are coarse, moderate and fine. Increasing the radiation resolution will lead to a higher number of directions and hence improved angular discretization of the radiative problem (usually a more accurate result).
To read more about supported types of radiation and radiation behavior check out this documentation page.
Last updated: June 15th, 2023
We appreciate and value your feedback.
Sign up for SimScale
and start simulating now